a 4 digit number is formed by repeating|A four digit number is formed by repeating a \\[2\\] digit number such : Cebu Question. A 4 - digit number is of the formed by repeating a 2 - digit number such as 2525, 3232 etc. Any number of the form is exactly divisible by : Solution. Formation of the number : Let the unit digit be x and the tens digit be y. So a 4 - digit . Kelebihan dan Kekurangan Mangoai.co Wifi Password Kelebihan dari mangoai.co meliputi keamanan data, kualitas informasi yang terjamin, harga terjangkau, serta legalitas yang jelas.Namun, beberapa kekurangan seperti keterbatasan dalam cakupan informasi perlu diperhatikan oleh pengguna.. Kesimpulan Dari ulasan ini, dapat .
a 4 digit number is formed by repeating,Question. A 4 - digit number is of the formed by repeating a 2 - digit number such as 2525, 3232 etc. Any number of the form is exactly divisible by : Solution. Formation of the number : Let the unit digit be x and the tens digit be y. So a 4 - digit .
Solution. Verified by Toppr. Let the unit digit be x and ten's digit be y. ∴ N umber = 1000y+100x+10y+x. ⇒ 1010y+101x. ⇒ 101(10y+x) So this number is divisible by .a 4 digit number is formed by repeating A four digit number is formed by repeating a \\[2\\] digit number suchA 4 digit number is formed by repeating a 2 digit number such as 2525, 3232 etc. Any number of this form is always exactly divisible by. 7; 11; 13; Smallest 3 digit prime numberA 4 digit number is formed by repeating a 2 digit number such as 2525, 3232, etc. Any number of this form is always exactly divisible is: 7 only. 11 only. 13 only. Smallest 3 . Hint: We have to find the number which always divides the number of the form of a four digit number which has a repeating \[2\] digit number like \[2525\], \[3232\] .
Step by step video, text & image solution for A 4-digit number is formed by repeating a 2 -digit number such as 1515, 3737 , etc . Any number of this form is .
a 4 digit number is formed by repeatingA 4 -digit number is formed by repeating a 2 -digit number such as 2525, 3232 etc. Any number of this form is exactly divisible by: Let D be a recurring decimal of the form D = . "A 4-digit number is formed by repeating a 2-digit number such as 2525, 3232 etc. Any number of this form is exactly divisible by 7 (b) 11 (c) 13 (d) Smalle.Question. A 4− digit number is formed by repeating a 2− digit number such as 2525,3232 etc. Any number of this from is exactly divisible by. 7. B. 11. C. 13. D. .A four digit number is formed by repeating a \\[2\\] digit number such A repeating integer is one in which a sequence of digits occurs two or more times to make the entire number. The 4-digit number 4242 is a repeating integer. How .
The required number of ways for this case = 2 × 4! = 48 Adding the number of ways in the two cases, 5 digit numbers that are divisible by 6 = 108 Required Probability = 108600 = 0.18 = 18 %A four-digit number is formed by using only the digits 1, 2 and 3 such that both 2 and 3 appear at least once. The number of all such four-digit numbers is - The question asks for the number of 4 digit numbers .How many 3-digit numbers can be formed from the digits 2, 3, 5, 8 and 9, without repetition, which are exactly divisible by 4? View Solution. Q5. How many different numbers of six digits (without repetition of digit) can be formed from the digits 3, 1, 7, 0, 9, 5?
A five digit number is formed by the digits 1, 2, 3, 4, 5 without repetition. Find the probability that the number is divisible by 4.
A 6-digit number is formed by repeating a 3-digit number; for example, 256256 or 678678 etc. Concept used: When we multiply 7, 11 and 13, it is equal to 1001. When any 3 digit number multiplied by 1001, it repeats twice. Calculation: We can write the given numbers in the following forms, ⇒ 256256 = 256 × 1001. ⇒ 678678 = 678 × 1001.
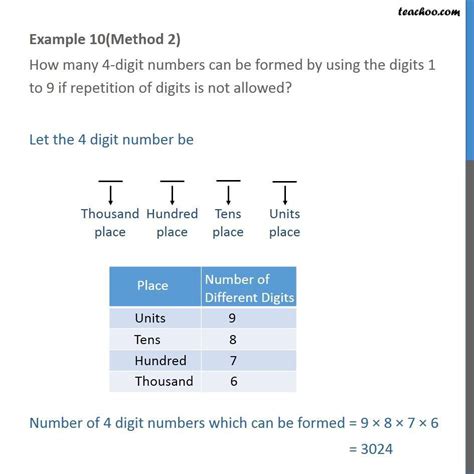
So, required number of ways in which four digit numbers can be formed from the given digits is 9 × 9 × 8 × 7 = 4536 Alternative Method: The thousands place of the 4-digit number is to be filled with any of the digits from 1 to 9 as the digit 0 cannot be included. Therefore, the number of ways in which thousands place can be filled is 9. A 4 digits number is formed using 2,3,5,7 and 9 without repeat. How many 4 digit numbers are there if each number has a remainder of 2 when divided either by 3 or 5? As i know, 2,3,5,7 and 9 is combination(No repetition) as order does not matter. But I don't understand the part of each number has remainder of 2 when divided either by 3 .Finding 6-digit numbers divisible by 10 formed by using the digits 0, 1, 3, 5, 7 and 9 when no digit is repeated. a number is divisible by 10 then 0 is at the unit place. _ _ _ _ _ 0 So, remaining digits are 1, 3, 5, 7 and 9 n = 5 and r = 5 ∴ Number of 6-digit number divisible by 10 = 5 P 5 = 5! (5 − 5)! = 5 × 4 × 3 × 2 × 1 0! = 120 .Click here:point_up_2:to get an answer to your question :writing_hand:a five digit number is formed by the digits 12345 with no digit repeated theThe tens place can now be filled by any of the remaining 5 digits (2, 3, 6, 7, 9). So, there are 5 ways of filling the tens place. The hundreds place can now be filled by any of the remaining 4 digits. So, there are 4 ways of filling it. ∴ .
Question 4: How many 4 – digit even numbers can be formed using the digits (3,5,7,9,1,0) if repetition of digits is not permitted? Answer: For even number unit digit must be 0, Now the remaining digits are 5 i.e., 3,5,7,9,1 now for the thousand place we have 5 option for the hundredth place we have 4 option and for the tens place we .

There are three odd digits, namely $$3, 5, 3$$ out of which $$3$$ occurs twice and $$5$$ occurs once. Number of ways to fill the odd places $$= \dfrac {3!}{2!} = 3$$. There are four even digits namely $$2, 4, 4, 2$$ out of which $$2$$ occurs twice and $$4$$ occurs twice.Here total number of digits = 10. Number of digits used (no digit is repeated) = 4. Since 0 cannot be filled in the fourth place, number of permutations for fourth place = 9. Now the remaining three places can be filled with 9 digits. ∴ Number of permutations = 9 P 3. 9! 6! = 9 × 8 × 7 × 6! 6! = 504. Hence total number of permutations = 9 .

The sum of all numbers greater than 1000 formed by using the digits 1, 3, 5, 7 such that no digit is being repeated in any number is. View Solution. Q4. Find the sum of all 4 digit numbers that can be formed using the digits 1, 3, 5, . Question 4: The number of six-digit numbers that can be established from the digits 1,2,3,4,5,6 and 7 so that digits do not repeat and the last digits are even is. Solution: Since, last digits are even. Therefore, For 1st place can be permeate in 3 ways and last place can be permeate in 2 ways and remaining places can be permeate in . 5 .A 4 digit number is formed by repeating a 2 digit number such as 2525, 3232 etc. Any number of this form is exactly divisible by. View Solution. Q5. Explain how they work? Write down any three-digit number(for example, 425). Make a six-digit number by repeating these digits in the same order (425425).
If 4 digit numbers greater than 5000 or randomly formed from the digits 0 ,1 ,3 ,5 and 7 what is the probability of forming a number divisible by 5 when:1) the digits repeated 2)the digits repetation is not allowed . Please explain the .
4-Digit Numbers Sum. The 4-digit numbers less than 2000 formed by the digits 1, 2, 3, and 4 where none of the digits is repeated are 1234, 1243, 1324, 1342, 1423, and 1432. Adding these numbers together gives the sum: 1234 + 1243 + 1324 + 1342 + 1423 + 1432 = 7998. So, the correct answer is (a) 7998.
a 4 digit number is formed by repeating|A four digit number is formed by repeating a \\[2\\] digit number such
PH0 · [Solved] A 4 digit number is formed by repeating a 2 digit number
PH1 · Solution: How many 4 digit numbers can be formed without
PH2 · Repeating integers question
PH3 · A four digit number is formed by repeating a \\[2\\] digit number such
PH4 · A four digit number is formed by repeating a \\[2\\] digit number
PH5 · A 4digit number is formed by repeating a 2digit number such as
PH6 · A 4 digit number is of the formed by repeating a 2 digit number such as
PH7 · A 4 digit number is of the formed by repeating a 2 digit
PH8 · A 4 digit number is formed by repeating a 2 digit number such
PH9 · A 4 digit number is formed by repeating a 2 digit number such
PH10 · A 4
PH11 · A 4
PH12 · "A 4